Code

Building Code Requirements for Structural Concrete (ACI 318-14) and Commentary (ACI 318R-14)

Reference

Reinforced Concrete Mechanics and Design, 6^th Edition, 2011, James Wight and James MacGregor, Pearson

Design Data

f_c’ = 5000 psi

f_y = 60,000 psi

Cover = 2.5 in. to the center of the reinforcement

Column 16 in. x 16 in.

Top reinforcement = 4 #9

Bottom reinforcement = 4 #9

Solution

Use the traditional hand calculations approach to generate the interaction diagram for the concrete column section shown above by determining the following seven control points:

Point 1: Pure compression

Point 2: Bar stress near tension face of member equal to zero, ( f_s = 0 )

Point 3: Bar stress near tension face of member equal to 0.5 f_y ( f_s = - 0.5 f_y )

Point 4: Bar stress near tension face of member equal to f_y ( f_s = - f_y )

Point 5: Bar strain near tension face of member equal to 0.005

Point 6: Pure bending

Point 7: Pure tension

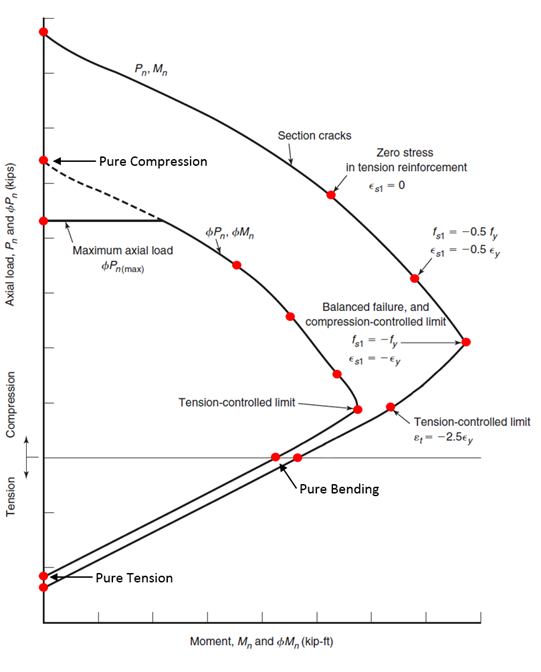
Figure 2 – Control Points

1. Pure Compression

1.1. Nominal axial compressive strength at zero eccentricity

ACI 318-14 (22.4.2.2)

1.2. Factored axial compressive strength at zero eccentricity

Since this column is a tied column with steel strain in compression:

ACI 318-14 (Table 21.2.2)

1.3. Maximum (allowable) factored axial compressive strength

ACI 318-14 (Table 22.4.2.1)

2. Bar Stress Near Tension Face of Member Equal to Zero, ( ε_s = f_s = 0 )

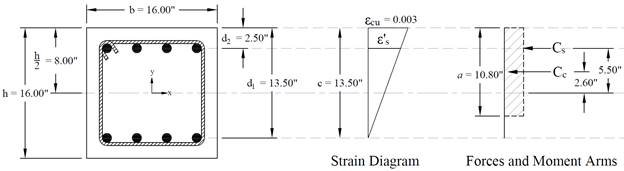
Figure 3 – Strains, Forces, and Moment Arms (ε_t = f_s = 0)

Strain ε_s is zero in the extreme layer of tension steel. This case is considered when calculating an interaction diagram because it marks the change from compression lap splices being allowed on all longitudinal bars, to the more severe requirement of tensile lap splices. ACI 318-14 (10.7.5.2.1 and 2)

2.1. c, a, and strains in the reinforcement

Where c is the distance from the fiber of maximum compressive strain to the neutral axis.

ACI 318-14 (22.2.2.4.2)

ACI 318-14 (22.2.2.4.1)

Where:

a = Depth of equivalent rectangular stress block

[IMA1] ACI 318-14 (Table 22.2.2.4.3)

ACI 318-14 (Table 21.2.2)

ACI 318-14 (22.2.2.1)

2.2. Forces in the concrete and steel

ACI 318-14 (22.2.2.4.1)

The area of the reinforcement in this layer has been included in the area (ab) used to compute C_c. As a result, it is necessary to subtract 0.85f_c’ from f_s’ before computing C_s:

2.3. ϕP_n and ϕM_n

3. Bar Stress Near Tension Face of Member Equal to 0.5 f_y, ( f_s = - 0.5 f_y )

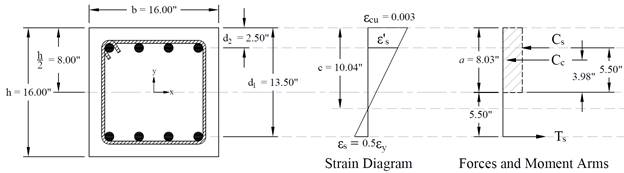
Figure 4 – Strains, Forces, and Moment Arms (f_s = - 0.5 f_y)

3.1. c, a, and strains in the reinforcement

ACI 318-14 (Table 21.2.2)

ACI 318-14 (22.2.2.1)

Where c is the distance from the fiber of maximum compressive strain to the neutral axis.

ACI 318-14 (22.2.2.4.2)

ACI 318-14 (22.2.2.4.1)

Where:

ACI 318-14 (Table 22.2.2.4.3)

3.2. Forces in the concrete and steel

ACI 318-14 (22.2.2.4.1)

The area of the reinforcement in this layer has been included in the area (ab) used to compute C_c. As a result, it is necessary to subtract 0.85f_c’ from f_s’ before computing C_s:

3.3. ϕP_n and ϕM_n

4. Bar Stress Near Tension Face of Member Equal to f_y, ( f_s = - f_y )

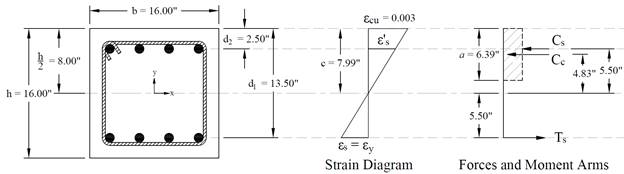
Figure 5 – Strains, Forces, and Moment Arms (f_s = - f_y)

This strain distribution is called the balanced failure case and the compression-controlled strain limit. It marks the change from compression failures originating by crushing of the compression surface of the section, to tension failures initiated by yield of longitudinal reinforcement. It also marks the start of the transition zone for ϕ for columns in which ϕ increases from 0.65 (or 0.75 for spiral columns) up to 0.90.

4.1. c, a, and strains in the reinforcement

ACI 318-14 (Table 21.2.2)

ACI 318-14 (22.2.2.1)

Where c is the distance from the fiber of maximum compressive strain to the neutral axis.

ACI 318-14 (22.2.2.4.2)

ACI 318-14 (22.2.2.4.1)

Where:

ACI 318-14 (Table 22.2.2.4.3)

4.2. Forces in the concrete and steel

ACI 318-14 (22.2.2.4.1)

The area of the reinforcement in this layer has been included in the area (ab) used to compute C_c. As a result, it is necessary to subtract 0.85f_c’ from f_s’ before computing C_s:

4.3. ϕP_n and ϕM_n

5. Bar Strain Near Tension Face of Member Equal to 0.005 in./in., ( ε_s = - 0.005 in./in.)

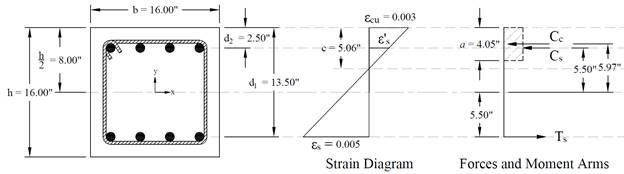
Figure 6 – Strains, Forces, and Moment Arms (ε_s = - 0.005 in./in.)

This corresponds to the tension-controlled strain limit of 0.005. It is the strain at the tensile limit of the transition zone for ϕ, used to define a tension-controlled section.

5.1. c, a, and strains in the reinforcement

ACI 318-14 (Table 21.2.2)

ACI 318-14 (22.2.2.1)

Where c is the distance from the fiber of maximum compressive strain to the neutral axis.

ACI 318-14 (22.2.2.4.2)

ACI 318-14 (22.2.2.4.1)

Where:

ACI 318-14 (Table 22.2.2.4.3)

5.2. Forces in the concrete and steel

ACI 318-14 (22.2.2.4.1)

The area of the reinforcement in this layer has been included in the area (ab) used to compute C_c. As a result, it is necessary to subtract 0.85f_c’ from f_s’ before computing C_s:

5.3. ϕP_n and ϕM_n

6. Pure Bending

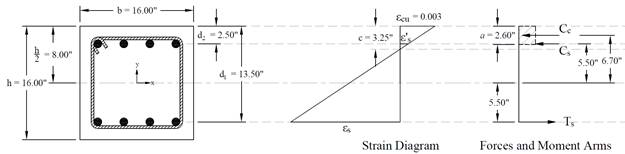
Figure 7 – Strains, Forces, and Moment Arms (Pure Moment)

This corresponds to the case where the nominal axial load capacity, P_n, is equal to zero. Iterative procedure is used to determine the nominal moment capacity as follows:

6.1. c, a, and strains in the reinforcement

Where c is the distance from the fiber of maximum compressive strain to the neutral axis.

ACI 318-14 (22.2.2.4.2)

ACI 318-14 (22.2.2.4.1)

Where:

ACI 318-14 (Table 22.2.2.4.3)

ACI 318-14 (22.2.2.1)

ACI 318-14 (Table 21.2.2)

6.2. Forces in the concrete and steel

ACI 318-14 (22.2.2.4.1)

The area of the reinforcement in this layer has been included in the area (ab) used to compute C_c. As a result, it is necessary to subtract 0.85f_c’ from f_s’ before computing C_s:

6.3. ϕP_n and ϕM_n

The assumption that c = 3.25 in. is correct

7. Pure Tension

The final loading case to be considered is concentric axial tension. The strength under pure axial tension is computed by assuming that the section is completely cracked through and subjected to a uniform strain greater than or equal to the yield strain in tension. The strength under such a loading is equal to the yield strength of the reinforcement in tension.

7.1. P_nt and ϕP_nt

ACI 318-14 (22.4.3.1)

ACI 318-14 (Table 21.2.2)

7.2. M_n and ϕM_n

Since the section is symmetrical

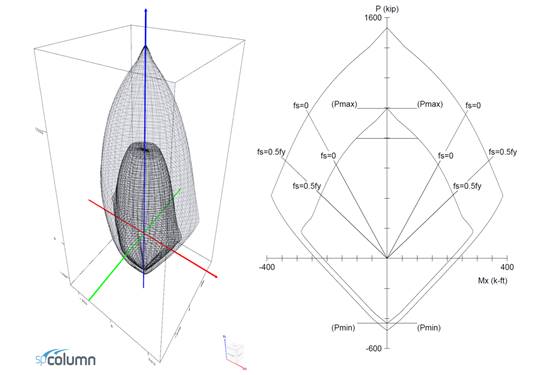
8. Column Interaction Diagram - spColumn Software

spColumn program performs the analysis of the reinforced concrete section conforming to the provisions of the Strength Design Method and Unified Design Provisions with all conditions of strength satisfying the applicable conditions of equilibrium and strain compatibility. For this column section, we ran in investigation mode with control points using the 318-14. In lieu of using program shortcuts, spSection (Figure 9) was used to place the reinforcement and define the cover to illustrate handling of irregular shapes and unusual bar arrangement.

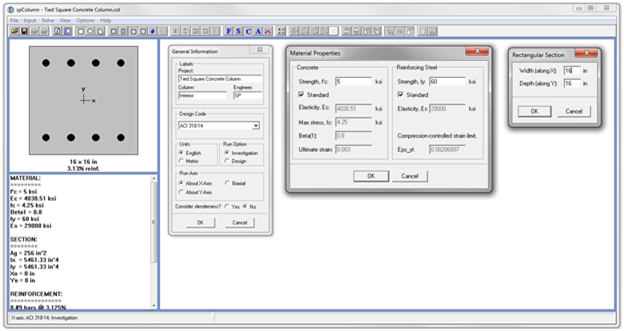
Figure 8 – Generating spColumn Model

Figure 9 – spColumn Model Editor (spSection)

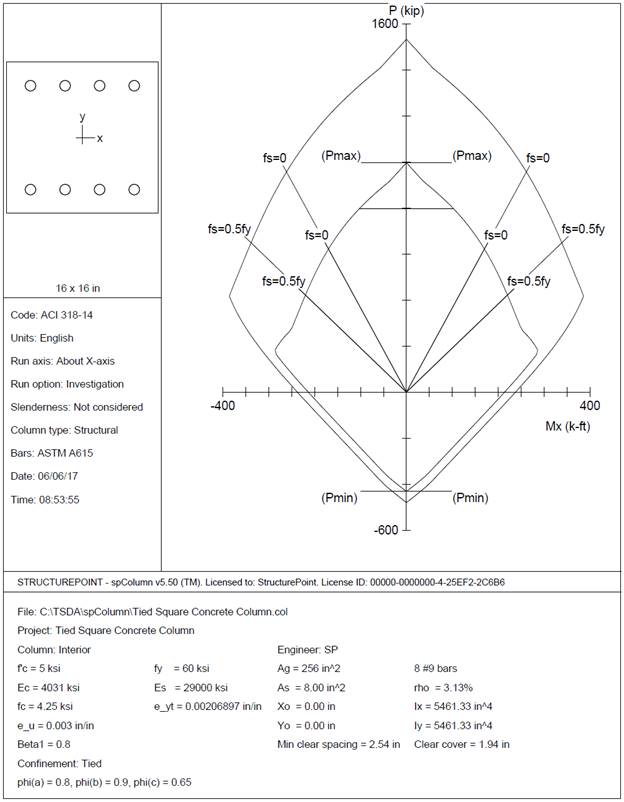
Figure 10 – Column Section Interaction Diagram about the X-Axis (spColumn)

9. Summary and Comparison of Design Results

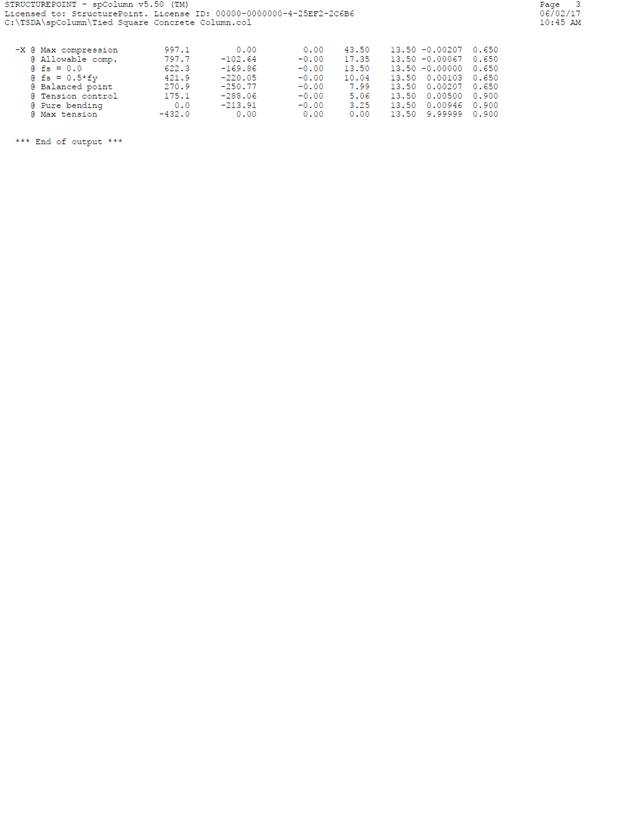
Table 1 - Comparison of Results
Support	*ϕP_n,* kip			*ϕM_n,* kip.ft
Support	Hand	Reference^*	spColumn	Hand	Reference^*	spColumn
Max compression	997	997	997	0	0	0
Allowable compression	798	798	798	---	---	---
f_s = 0.0	622	622	622	170	170	170
f_s = 0.5 f_y	422	422	422	220	220	220
Balanced point	271	271	271	251	251	251
Tension control	175	175	175	288	288	288
Pure bending	0	0	0	214	214	214
Max tension	432	432	432	0	0	0
^* Reinforced Concrete Mechanic and Design, 6^th Edition, James Wight & MacGregor – Example 11-1

In all of the hand calculations and the reference used illustrated above, the results are in precise agreement with the automated exact results obtained from the spColumn program.

10. Conclusions & Observations

The analysis of the reinforced concrete section performed by spColumn conforms to the provisions of the Strength Design Method and Unified Design Provisions with all conditions of strength satisfying the applicable conditions of equilibrium and strain compatibility.

In the calculation shown above a P-M interaction diagram was generated with moments about the X-Axis (Uniaxial bending). Since the reinforcement in the section is not symmetrical, a different P-M interaction diagram is needed for the other orthogonal direction about the Y-Axis (See the following Figure for the case where f_s = f_y).

Figure 11 – Strains, Forces, and Moment Arms (f_s = - f_y Moments About x- and y-axis)

When running about the Y-Axis, we have 2 bars in 4 layers instead of 4 bars in just 2 layers (about X-Axis) resulting in a completely different interaction diagram as shown in the following Figure.

Figure 12 – Comparison of Column Interaction Diagrams about X-Axis and Y-Axis (spColumn)

Further differences in the interaction diagram in both directions can result if the column cross section geometry is irregular.

In most building design calculations, such as the examples shown for flat plate or flat slab concrete floor systems, all building columns are subjected to M_x and M_y due to lateral forces and unbalanced moments from both directions of analysis. This requires an evaluation of the column P-M interaction diagram in two directions simultaneously (biaxial bending).

StucturePoint’s spColumn program can also evaluate column sections in biaxial mode to produce the results shown in the following Figure for the column section in this example.

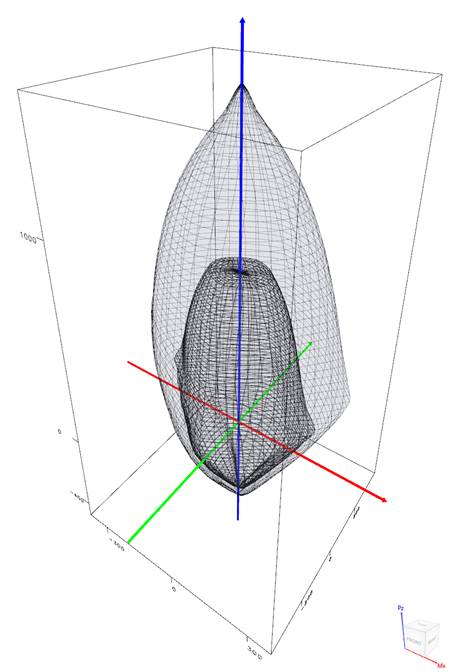
Figure 13 – Nominal & Design Interaction Diagram in Two Directions (Biaxial) (spColumn)

2. Bar Stress Near Tension Face of Member Equal to Zero, ( εs = fs = 0 )

2.3. ϕPn and ϕMn

3. Bar Stress Near Tension Face of Member Equal to 0.5 fy, ( fs = - 0.5 fy )

3.3. ϕPn and ϕMn

4. Bar Stress Near Tension Face of Member Equal to fy, ( fs = - fy )

4.3. ϕPn and ϕMn

5. Bar Strain Near Tension Face of Member Equal to 0.005 in./in., ( εs = - 0.005 in./in.)

5.3. ϕPn and ϕMn

6.3. ϕPn and ϕMn

7.1. Pnt and ϕPnt

7.2. Mn and ϕMn

8. Column Interaction Diagram - spColumn Software

9. Summary and Comparison of Design Results

2. Bar Stress Near Tension Face of Member Equal to Zero, ( ε_s = f_s = 0 )

2.3. ϕP_n and ϕM_n

3. Bar Stress Near Tension Face of Member Equal to 0.5 f_y, ( f_s = - 0.5 f_y )

3.3. ϕP_n and ϕM_n

4. Bar Stress Near Tension Face of Member Equal to f_y, ( f_s = - f_y )

4.3. ϕP_n and ϕM_n

5. Bar Strain Near Tension Face of Member Equal to 0.005 in./in., ( ε_s = - 0.005 in./in.)

5.3. ϕP_n and ϕM_n

6.3. ϕP_n and ϕM_n

7.1. P_nt and ϕP_nt

7.2. M_n and ϕM_n