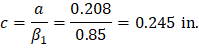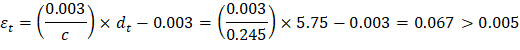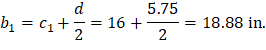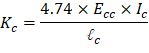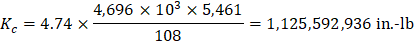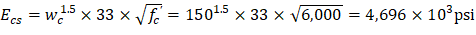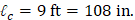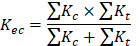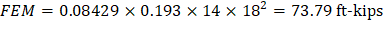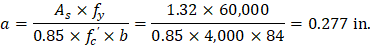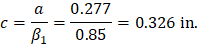3. Two-Way Slab Analysis and Design
ACI 318 states that a slab system shall be designed by any procedure satisfying equilibrium and geometric compatibility, provided that strength and serviceability criteria are satisfied. Distinction of two-systems from one-way systems is given by ACI 318-14 (R8.10.2.3 & R8.3.1.2).
ACI 318 permits the use of Direct Design Method (DDM) and Equivalent Frame Method (EFM) for the gravity load analysis of orthogonal frames and is applicable to flat plates, flat slabs, and slabs with beams. The following sections outline the solution per DDM, EFM, and spSlab software respectively.
3.1. Direct Design Method (DDM)
Two-way slabs satisfying the limits in ACI 318-14 (8.10.2) are permitted to be designed in accordance with the DDM.
3.1.1. Direct Design Method Limitations
There is a minimum of three continuous spans in each direction | ACI 318-14 (8.10.2.1) |
Successive span lengths are equal | ACI 318-14 (8.10.2.2) |
Long-to-short span ratio is 1.29 < 2 | ACI 318-14 (8.10.2.3) |
Columns are not offset | ACI 318-14 (8.10.2.4) |
Loads are uniformly distributed over the entire panel | ACI 318-14 (8.10.2.5) |
Service live-to-dead load ratio of 0.37 < 2.0 | ACI 318-14 (8.10.2.6) |
Slab system is without beams and this requirement is not applicable | ACI 318-14 (8.10.2.7) |
Since all the criteria are met, Direct Design Method can be utilized. | |
a) Calculate the total factored static moment:
ACI 318-14 (8.10.3.2) | |
b) Distribute the total factored moment, Mo, in an interior and end span: | ACI 318-14 (8.10.4) |
Table 1 - Distribution of Mo along the span | ||
Location | Total Design Strip Moment, | |
Exterior Span | Exterior Negative | 0.26 × Mo = 24.39 |
Positive | 0.52 × Mo = 48.79 | |
Interior Negative | 0.70 × Mo = 65.67 | |
Interior Span | Positive | 0.35 × Mo = 32.84 |
c) Calculate the column strip moments. | ACI 318-14 (8.10.5) |
That portion of negative and positive total design strip moments not resisted by column strips shall be proportionally assigned to corresponding two half-middle strips. | ACI 318-14 (8.10.6.1) |
Table 2 - Lateral Distribution of the Total Design Strip Moment, MDS | ||||
Location | Total Design Strip | Column Strip | Moment in Two | |
Exterior Span | Exterior Negative* | 24.39 | 1.00 × MDS = 24.39 | 0.00 × MDS = 0.00 |
Positive | 48.79 | 0.60 × MDS = 29.27 | 0.40 × MDS = 19.52 | |
Interior Negative* | 65.67 | 0.75 × MDS = 49.25 | 0.25 × MDS = 16.42 | |
Interior Span | Positive | 32.84 | 0.60 × MDS = 19.70 | 0.40 × MDS = 13.14 |
* All negative moments are at face of support. | ||||
3.1.3. Flexural Reinforcement Requirements
a) Determine flexural reinforcement required for column and middle strips at all critical sections
The following calculation is for the exterior span exterior negative location of the column strip.
Mu = 24.39 ft-kips
Use average davg = 5.75 in.
To determine the area of steel, assumptions have to be made whether the section is tension or compression controlled, and regarding the distance between the resultant compression and tension forces along the slab section (jd). In this example, tension-controlled section will be assumed so the reduction factor ϕ is equal to 0.9, and jd will be taken equal to 0.95d. The assumptions will be verified once the area of steel in finalized.
Assume jd = 0.95 × d = 5.46 in.
Column strip width, | ||
Middle strip width, |
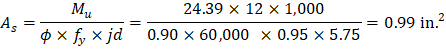
Recalculate ‘a’ for the actual As = 0.99 in.2
| |
| |
|
Therefore, the assumption that section is tension-controlled is valid.
ACI 318-14 (24.4.3.2) | |
Maximum spacing smax = 2 × h = 14 in. < 18 in. | ACI 318-14 (8.7.2.2) |
Provide 6 - #4 bars with As = 1.20 in.2 and |
Based on the procedure outlined above, values for all span locations are given in Table 3.
Mu | b | d | As,req (in.2) | As,min (in.2) | Reinforcement Provided | As,provided (in.2) | ||
End Span | ||||||||
Column Strip | Exterior Negative | 24.39 | 84 | 5.75 | 0.96 | 1.06 | 6 - #4 | 1.20 |
Positive | 29.27 | 84 | 5.75 | 1.16 | 1.06 | 6 - #4 | 1.20 | |
Interior Negative | 49.25 | 84 | 5.75 | 1.98 | 1.06 | 10 - #4 | 2.00 | |
Middle Strip | Exterior Negative | 0.00 | 84 | 5.75 | 0.00 | 1.06 | 6 - #4 | 1.20 |
Positive | 19.52 | 84 | 5.75 | 0.77 | 1.06 | 6 - #4 | 1.20 | |
Interior Negative | 16.42 | 84 | 5.75 | 0.64 | 1.06 | 6 - #4 | 1.20 | |
Interior Span | ||||||||
Column Strip | Positive | 19.70 | 84 | 5.75 | 0.77 | 1.06 | 6 - #4 | 1.20 |
Middle Strip | Positive | 13.14 | 84 | 5.75 | 0.51 | 1.06 | 6 - #4 | 1.20 |
b) Calculate additional slab reinforcement at columns for moment transfer between slab and column
The factored slab moment resisted by the column (γf × Mu) shall be assumed to be transferred by flexure. Concentration of reinforcement over the column by closer spacing or additional reinforcement shall be used to resist this moment. The fraction of slab moment not calculated to be resisted by flexure shall be assumed to be resisted by eccentricity of shear.
ACI 318-14 (8.4.2.3)
Portion of the unbalanced moment transferred by flexure is γf × Mu | ACI 318-14 (8.4.2.3.1) |
Where: | |
ACI 318-14 (8.4.2.3.2) |
b1 = Dimension of the critical section bo measured in the direction of the span for which moments are determined in ACI 318, Chapter 8 (see Figure 5). b2 = Dimension of the critical section bo measured in the direction perpendicular to b1 in ACI 318, Chapter 8 (see Figure 5). bb = Effective slab width = c2 + 3×h ACI 318-14 (8.4.2.3.3)
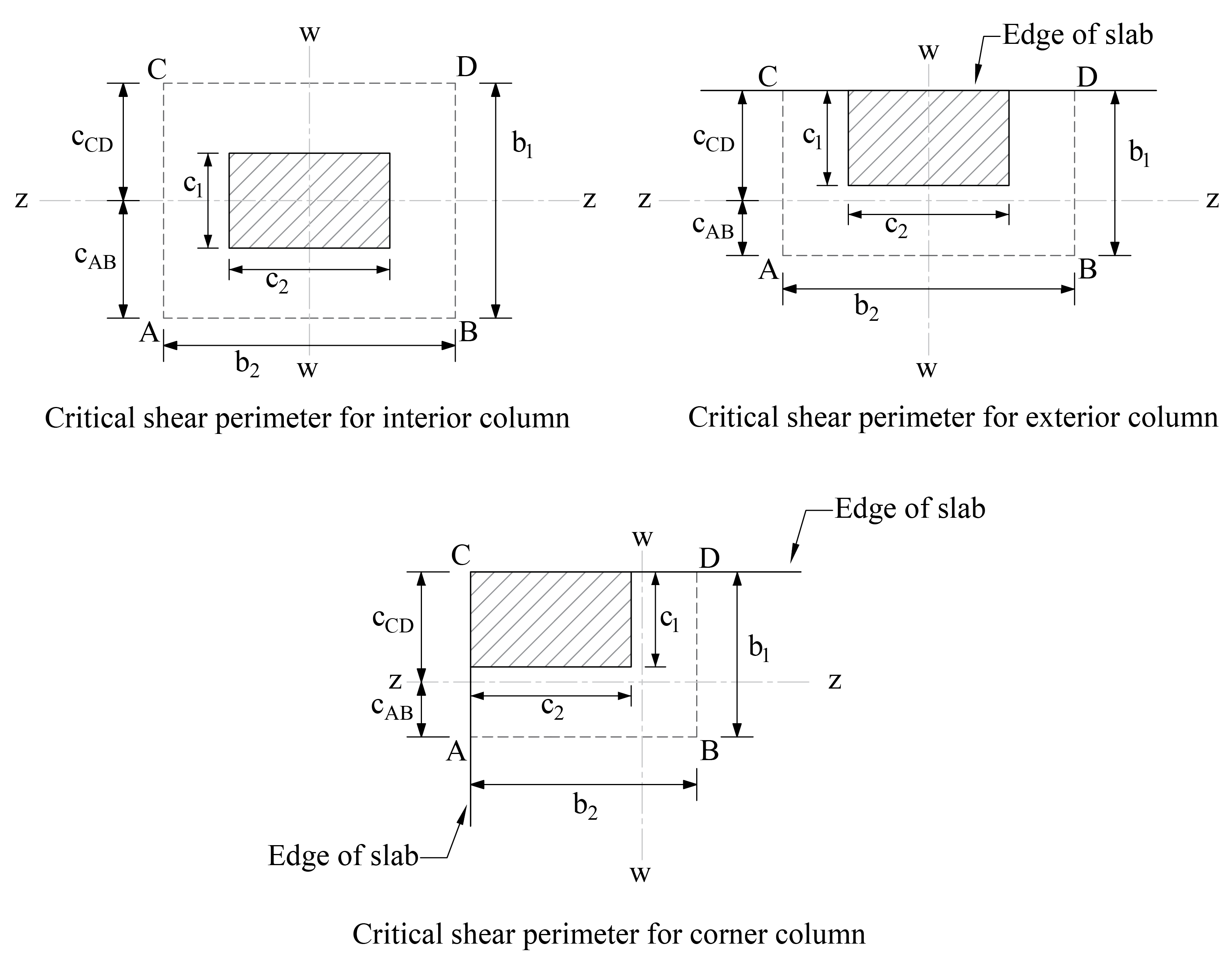
Figure 5 - Critical Shear Perimeters for Columns
The additional reinforcement calculation for the column strip of end span – exterior negative location is provided below.
Mu = 24.39 ft-kips | As(prov) = 1.20 in.2 |
| |
Where b = 84 in. is the column strip width
Provide 1 - #4 bars with As = 0.20 in.2
Table 4 - Additional Slab Reinforcement required for moment transfer between slab and column (DDM) | |||||||||
Span Location | Mu* (ft-kips) | γf | γf Mu (ft-kips) | Effective slab width, bb (in.) | d (in.) | As req’d within | As prov. For flexure | Add’l Reinf. | |
End Span | |||||||||
Column Strip | Exterior Negative | 24.39 | 0.62 | 15.10 | 37 | 5.75 | 0.60 | 0.53 | 1-#4 |
Interior Negative | 0.00 | 0.60 | 0.00 | 37 | 5.75 | 0.00 | 0.97 | - | |
* Mu is taken at the centerline of the support in Equivalent Frame Method solution. | |||||||||
3.1.4. Factored Moments in Columns
a) Interior columns:
ACI 318-14 (8.10.7.2) | |
With the same column size and length above and below the slab,
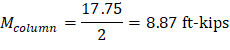
b) Exterior Columns:
Total exterior negative moment from slab must be transferred directly to the column: Mu = 24.39 ft-kips. With the same column size and length above and below the slab,

The moments determined above are combined with the factored axial loads (for each story) for design of column sections as shown later in this example.
3.2. Equivalent Frame Method (EFM)
EFM is the most comprehensive and detailed procedure provided by the ACI 318 for the analysis and design of two-way slab systems where the structure is modeled by a series of equivalent frames (interior and exterior) on column lines taken longitudinally and transversely through the building.
The equivalent frame consists of three parts:
1) Horizontal slab-beam strip, including any beams spanning in the direction of the frame. Different values of moment of inertia along the axis of slab-beams should be taken into account where the gross moment of inertia at any cross section outside of joints or column capitals shall be taken, and the moment of inertia of the slab-beam at the face of the column, bracket or capital divide by the quantity (1-c2/l2)2 shall be assumed for the calculation of the moment of inertia of slab-beams from the center of the column to the face of the column, bracket or capital.
ACI 318-14 (8.11.3)
2) Columns or other vertical supporting members, extending above and below the slab. Different values of moment of inertia along the axis of columns should be taken into account where the moment of inertia of columns from top and bottom of the slab-beam at a joint shall be assumed to be infinite, and the gross cross section of the concrete is permitted to be used to determine the moment of inertia of columns at any cross section outside of joints or column capitals.
ACI 318-14 (8.11.4)
3) Elements of the structure (Torsional members) that provide moment transfer between the horizontal and vertical members. These elements shall be assumed to have a constant cross section throughout their length consisting of the greatest of the following: (1) portion of slab having a width equal to that of the column, bracket, or capital in the direction of the span for which moments are being determined, (2) portion of slab specified in (1) plus that part of the transverse beam above and below the slab for monolithic or fully composite construction, (3) the transverse beam includes that portion of slab on each side of the beam extending a distance equal to the projection of the beam above or below the slab, whichever is greater, but not greater than four times the slab thickness.
ACI 318-14 (8.11.4)
3.2.1. Equivalent Frame Method Limitations
In EFM, live load shall be arranged in accordance with 6.4.3 which requires slab systems to be analyzed and designed for the most demanding set of forces established by investigating the effects of live load placed in various critical patterns.
ACI 318-14 (8.111.2&6.4.3)
Complete analysis must include representative interior and exterior equivalent frames in both the longitudinal and transverse directions of the floor
ACI 318-14 (8.11.2.1)
Panels shall be rectangular, with a ratio of longer to shorter panel dimensions, measured center-to-center of supports, not to exceed 2.
ACI 318-14 (8.10.2.3)
3.2.2. Frame Members of Equivalent Frame
Determine moment distribution factors and fixed-end moments for the equivalent frame members. The moment distribution procedure will be used to analyze the equivalent frame. Stiffness factors k, carry over factors COF, and fixed-end moment factors FEM for the slab-beams and column members are determined using the design aids tables at Appendix 20A of PCA Notes on ACI 318-11.
These calculations are shown below.
a) Flexural stiffness of slab-beams at both ends, Ksb
, | |
For cF1 = cF2, stiffness factors, kNF = kFN = 4.127 | PCA Notes on ACI 318-11 (Table A1) |
Thus, | PCA Notes on ACI 318-11 (Table A1) |
PCA Notes on ACI 318-11 (Table A1) | |
Where, | |
ACI 318-14 (19.2.2.1.a) | |
Carry-over factor COF = 0.507 | PCA Notes on ACI 318-11 (Table A1) |
Fixed-end moment | PCA Notes on ACI 318-11 (Table A1) |
b) Flexural stiffness of column members at both ends, Kc
Referring to Table A7, Appendix 20A,
ta = h/2 = 7/2 = 3.5 in. | tb = h/2 = 7/2 = 3.5 in. |
H = 9 ft = 108 in. | Hc = H - ta - tb = 108 – 3.5 – 3.5 = 101 in. |
Then,
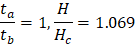
Thus, kAB = kBA = 4.74 by interpolation.
| PCA Notes on ACI 318-11 (Table A7) |
| |
Where | |
| ACI 318-14 (19.2.2.1.a) |
|
c) Torsional stiffness of torsional members, Kt.
PCA Notes on ACI 318-11 (Table A7) Where c2 = 16 in., and l2 = 14 ft = 168 in.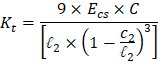
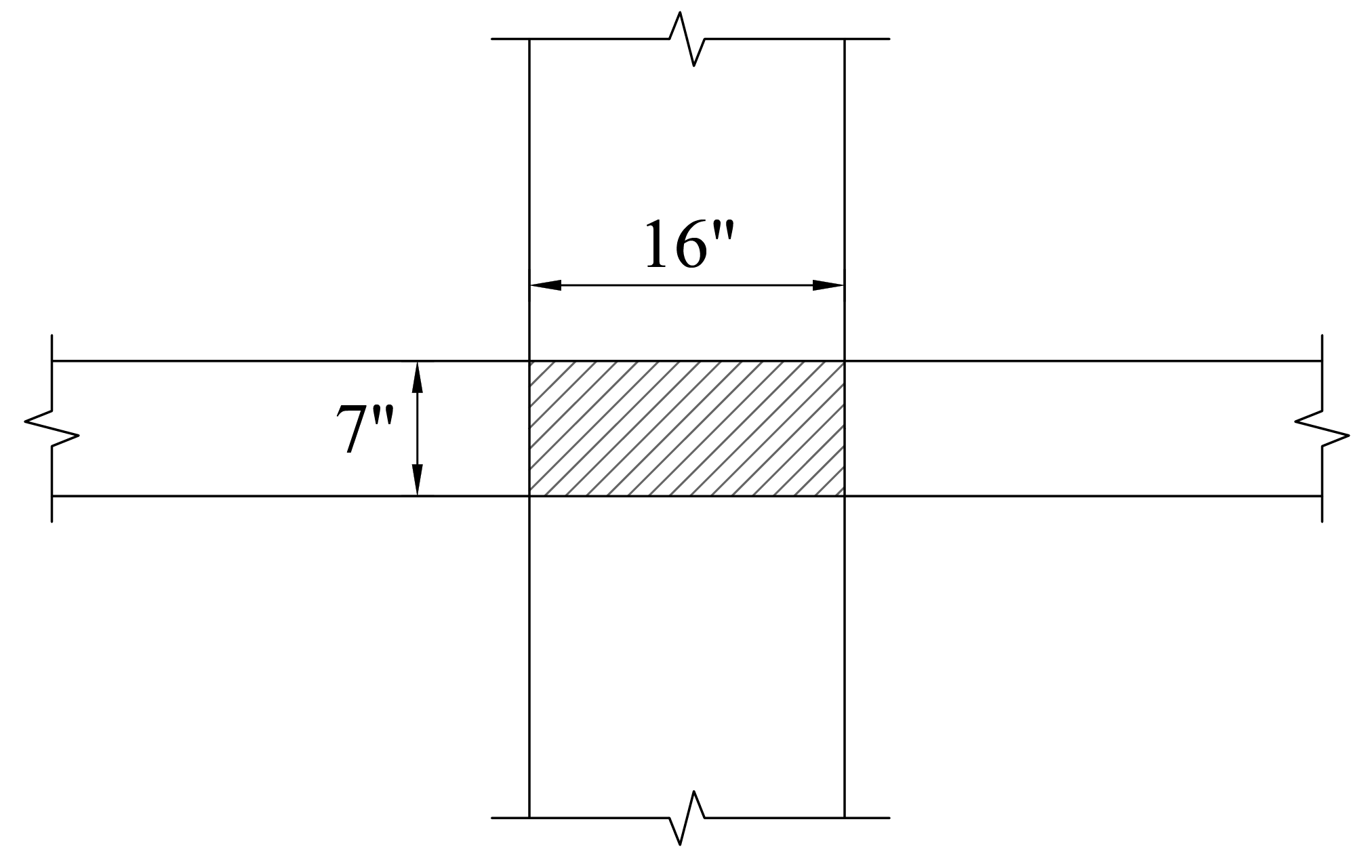
Figure 6 - Torsional Member
d) Equivalent column stiffness Kec.
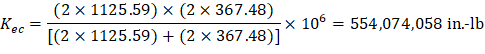
Where ΣKt is for two torsional members one on each side of the column, and ΣKc is for the upper and lower columns at the slab-beam joint of an intermediate floor.
Figure 7 - Column and Edge of Slab
e) Slab-beam joint distribution factors, DF.
At exterior joint At interior joint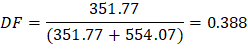

COF for slab-beam = 0.507

Figure 8 - Slab and Column Stiffness
3.2.3. Equivalent Frame Analysis
Determine negative and positive moments for the slab-beams using the moment distribution method. Since the unfactored live load does not exceed three-quarters of the unfactored dead load, design moments are assumed to occur at all critical sections with full factored live on all spans.
ACI 318-14 (6.4.3.2)

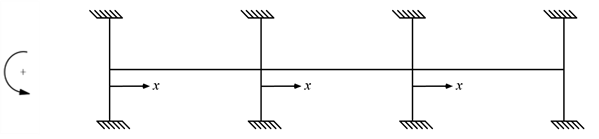
a) Factored load and Fixed-End Moments (FEM’s).
Factored dead load, Factored live load, ACI 318-14 (5.3.1) Total factored load,
FEM’s for slab-beams | PCA Notes on ACI 318-11 (Table A1) |
|
b) Moment distribution. Computations are shown in Table 5. Counterclockwise rotational moments acting on the member ends are taken as positive. Maximum positive span moments are determined from the following equation:
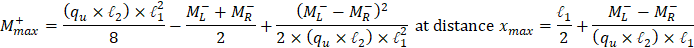
Where:
• Mmax+ = Maximum positive moment in the span • ML- = Negative moment in the left support • MR- = Negative moment in the right support • l1 = The span length
The reactions (shear forces) at supports are given by the following equations:
Where:
• VL = Reaction (shear force) at the left support • VR = Reaction (shear force) at the right support
Maximum positive moment in spans 1-2 and 3-4:
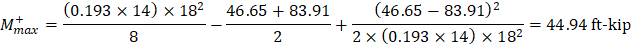
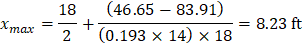
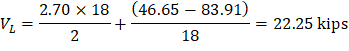
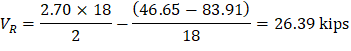
Where:
ML- = 46.65 ft-kip MR- = 83.95 ft-kip
Maximum positive moment in span 2-3:
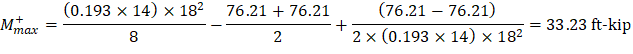
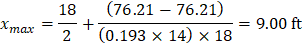
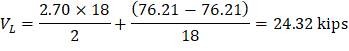
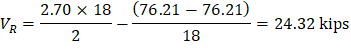
Where:
ML- = 76.21 ft-kip MR- = 76.21 ft-kip
Table 5 - Moment distribution for equivalent frame method (EFM) | ||||||
Joint | 1 | 2 | 3 | 4 | ||
Member | 1-2 | 2-1 | 2-3 | 3-2 | 3-4 | 4-3 |
DF | 0.388 | 0.280 | 0.280 | 0.280 | 0.280 | 0.388 |
COF | 0.507 | 0.507 | 0.507 | 0.507 | 0.507 | 0.507 |
FEM | 73.79 | -73.79 | 73.79 | -73.79 | 73.79 | -73.79 |
Dist | -28.66 | 0.00 | 0.00 | 0.00 | 0.00 | 28.66 |
CO | 0.00 | -14.53 | 0.00 | 0.00 | 14.53 | 0.00 |
Dist | 0.00 | 4.06 | 4.06 | -4.06 | -4.06 | 0.00 |
CO | 2.06 | 0.00 | -2.06 | 2.06 | 0.00 | -2.06 |
Dist | -0.80 | 0.58 | 0.58 | -0.58 | -0.58 | 0.80 |
CO | 0.29 | -0.41 | -0.29 | 0.29 | 0.41 | -0.29 |
Dist | -0.11 | 0.20 | 0.20 | -0.20 | -0.20 | 0.11 |
CO | 0.10 | -0.06 | -0.10 | 0.10 | 0.06 | -0.10 |
Dist | -0.04 | 0.04 | 0.04 | -0.04 | -0.04 | 0.04 |
CO | 0.02 | -0.02 | -0.02 | 0.02 | 0.02 | -0.02 |
Dist | -0.01 | 0.01 | 0.01 | -0.01 | -0.01 | 0.01 |
CO | 0.01 | 0.00 | -0.01 | 0.01 | 0.00 | -0.01 |
Dist | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
M-max | 46.65 | -83.91 | 76.21 | -76.21 | 83.91 | -46.65 |
V | 22.25 | 26.39 | 24.32 | 24.32 | 26.39 | 22.25 |
xmax | 8.23 | 9.00 | 9.77 | |||
M+max | 44.94 | 33.23 | 44.94 | |||
Positive and negative factored moments for the slab system in the direction of analysis are plotted in Figure 9. The negative moments used for design are taken at the faces of supports (rectangle section or equivalent rectangle for circular or polygon sections) but not at distances greater than 0.175×l1 from the centers of supports.
ACI 318-14 (8.11.6.1)
(use face of support location)
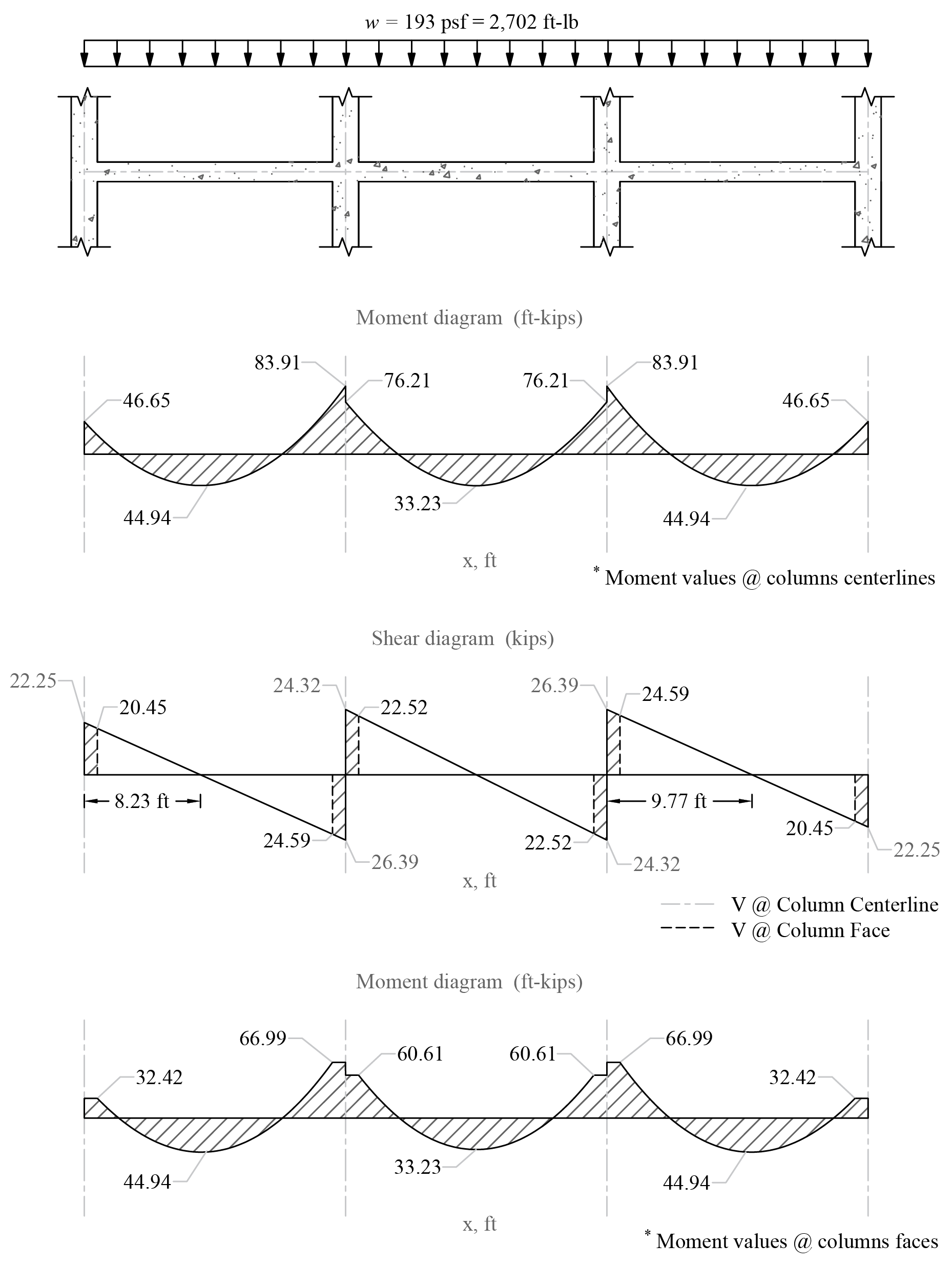
3.2.5. Distribution of Design Moments
a) Check whether the moments calculated above can take advantage of the reduction permitted by ACI 318-14 (8.11.6.5):
If the slab system analyzed using EFM within the limitations of ACI 318-14 (8.10.2), it is permitted by the ACI code to reduce the calculated moments obtained from EFM in such proportion that the absolute sum of the positive and average negative design moments need not exceed the value obtained from the following equation:
| ACI 318-14 (Eq. 8.10.3.2) |
End spans: | |
Interior span: |
The total design moments from the Equivalent Frame Method yield a static moment equal to that given by the Direct Design Method and no appreciable reduction can be realized.
b) Distribute factored moments to column and middle strips:
After the negative and positive moments have been determined for the slab-beam strip, the ACI code permits the distribution of the moments at critical sections to the column strips, beams (if any), and middle strips in accordance with the DDM.
ACI 318-14 (8.11.6.6)
Distribution of factored moments at critical sections is summarized in Table 6.
Slab-beam Strip | Column Strip | Middle Strip | ||||
Moment | Percent | Moment | Percent | Moment | ||
End Span | Exterior Negative | 32.42 | 100 | 32.42 | 0 | 0 |
Positive | 44.94 | 60 | 26.96 | 40 | 17.98 | |
Interior Negative | 66.99 | 75 | 50.24 | 25 | 16.75 | |
Interior Span | Negative | 60.61 | 75 | 45.46 | 25 | 15.15 |
Positive | 33.23 | 60 | 19.94 | 40 | 13.29 | |
3.2.6. Flexural Reinforcement Requirements
a) Determine flexural reinforcement required for column and middle strips at all critical sections
The following calculation is for the exterior span exterior negative location of the column strip.
Mu = 24.39 ft-kips
Use average davg = 5.75 in.
To determine the area of steel, assumptions have to be made whether the section is tension or compression controlled, and regarding the distance between the resultant compression and tension forces along the slab section (jd). In this example, tension-controlled section will be assumed so the reduction factor ϕ is equal to 0.9, and jd will be taken equal to 0.95d. The assumptions will be verified once the area of steel in finalized.
Assume jd = 0.95 × d = 5.46 in.
Column strip width, | ||
Middle strip width, |
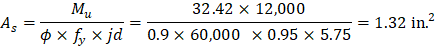
Recalculate ‘a’ for the actual As = 1.32 in.2
| |
| |
|
Therefore, the assumption that section is tension-controlled is valid.
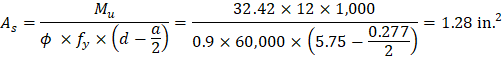
| ACI 318-14 (24.4.3.2) |
Maximum spacing smax = 2 × h = 14 in. < 18 in. | ACI 318-14 (8.7.2.2) |
Provide 6 - #4 bars with As = 1.40 in.2 and |
Based on the procedure outlined above, values for all span locations are given in Figure 9.
Mu b d As,req (in.2) As,min (in.2) Reinforcement Provided As,provided (in.2) End Span Column Strip Exterior Negative 32.42 84 5.75 1.28 1.06 7 - #4 1.40 Positive 26.96 84 5.75 1.06 1.06 6 - #4 1.20 Interior Negative 50.24 84 5.75 2.02 1.06 11 - #4 2.20 Middle Strip Exterior Negative 0.00 84 5.75 0.00 1.06 6 - #4 1.20 Positive 17.98 84 5.75 0.70 1.06 6 - #4 1.20 Interior Negative 16.75 84 5.75 0.66 1.06 6 - #4 1.20 Interior Span Column Strip Positive 19.94 84 5.75 0.78 1.06 6 - #4 1.20 Middle Strip Positive 13.29 84 5.75 0.52 1.06 6 - #4 1.20
(ft-kips)
(in.)
(in.)
b) Calculate additional slab reinforcement at columns for moment transfer between slab and column
The factored slab moment resisted by the column (γf × Mu) shall be assumed to be transferred by flexure. Concentration of reinforcement over the column by closer spacing or additional reinforcement shall be used to resist this moment. The fraction of slab moment not calculated to be resisted by flexure shall be assumed to be resisted by eccentricity of shear.
ACI 318-14 (8.4.2.3)
Portion of the unbalanced moment transferred by flexure is γf × Mu | ACI 318-14 (8.4.2.3.1) |
Where: | |
ACI 318-14 (8.4.2.3.2) |
b1 = Dimension of the critical section bo measured in the direction of the span for which moments are determined in ACI 318, Chapter 8 (see Figure 5). | |
b2 = Dimension of the critical section bo measured in the direction perpendicular to b1 in ACI 318, Chapter 8 (see Figure 5). | |
bb = Effective slab width = c2 + 3×h | ACI 318-14 (8.4.2.3.3) |
The additional reinforcement calculation for the column strip of end span – exterior negative location is provided below.
Mu = 46.65 ft-kips | As(prov) = 1.40 in.2 |
| |
| |
Where b = 84 in. is the column strip width
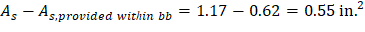
Provide 3- #4 bars with As = 0.60 in.2
Table 8 - Additional Slab Reinforcement required for moment transfer between slab and column (EFM) | |||||||||
Span Location | Mu* (ft-kips) | γf | γf Mu (ft-kips) | bb (in.) | d (in.) | As req’d within | As prov. For flexure | Add’l Reinf. | |
End Span | |||||||||
Column Strip | Exterior Negative | 46.65 | 0.62 | 28.78 | 37 | 5.75 | 1.17 | 0.62 | 3-#4 |
Interior Negative | 7.71 | 0.60 | 4.63 | 37 | 5.75 | 0.18 | 0.97 | - | |
* Mu is taken at the centerline of the support in Equivalent Frame Method solution. | |||||||||
The unbalanced moment from the slab-beams at the supports of the equivalent frame are distributed to the support columns above and below the slab-beam in proportion to the relative stiffness of the support columns. Referring to Figure 9, the unbalanced moment at joints 1 and 2 are:
Joint 1= + 46.65 ft-kips
Joint 2= -83.91 + 76.21 = -7.71 ft-kips
The stiffness and carry-over factors of the actual columns and the distribution of the unbalanced slab moments to the exterior and interior columns are shown in the Figure 10.
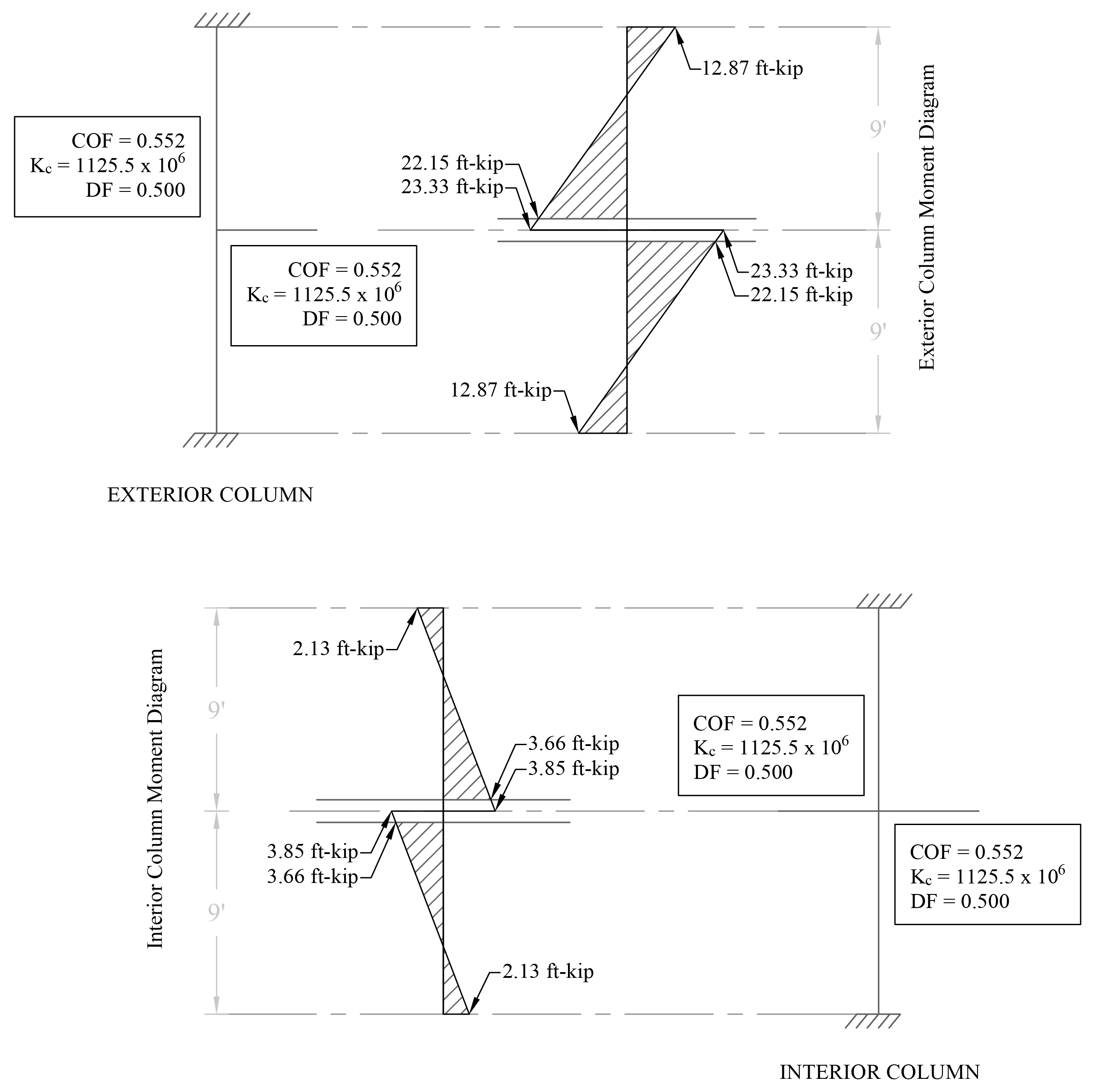
Figure 10 - Column Moments (Unbalanced Moments from Slab-Beam)
Mcol,Exterior = 22.15 ft-kips
Mcol,Interior = 3.66 ft-kips
The moments determined above are combined with the factored axial loads (for each story) and factored moments in the transverse direction for design of column sections. The Figure 11 shows the moment diagrams in the longitudinal and transverse direction for the interior and exterior equivalent frames. Following the previous procedure, the moment values at the face of interior, exterior, and corner columns from the unbalanced moment values can be obtained. These values are shown in the following table.
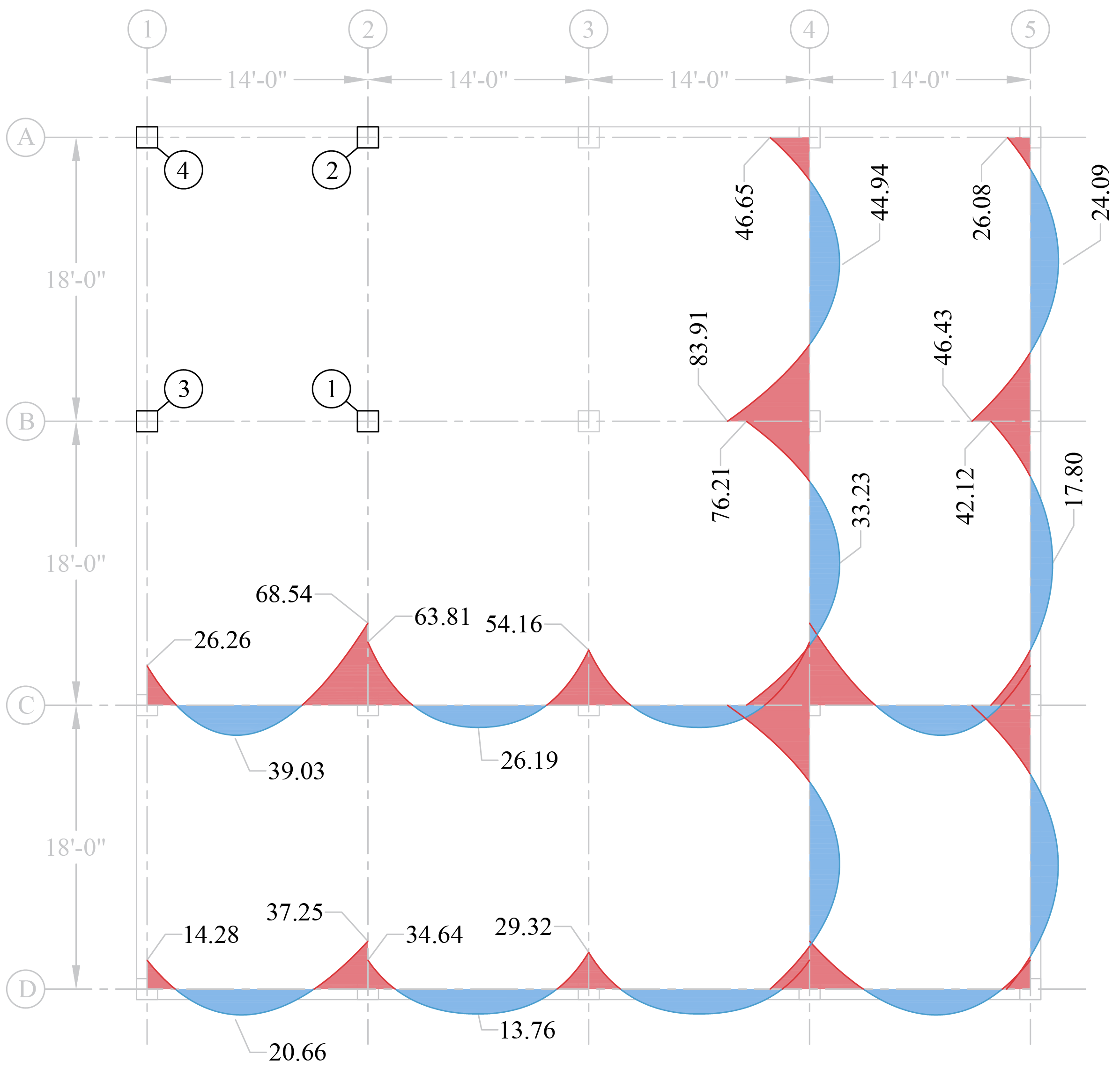
Figure 11 - Moment Diagrams (kips-ft)
Mu | Column number (See the previous Figure) | |||
1 | 2 | 3 | 4 | |
Mux | 3.66 | 22.15 | 2.05 | 12.47 |
Muy | 2.27 | 1.24 | 12.26 | 6.78 |