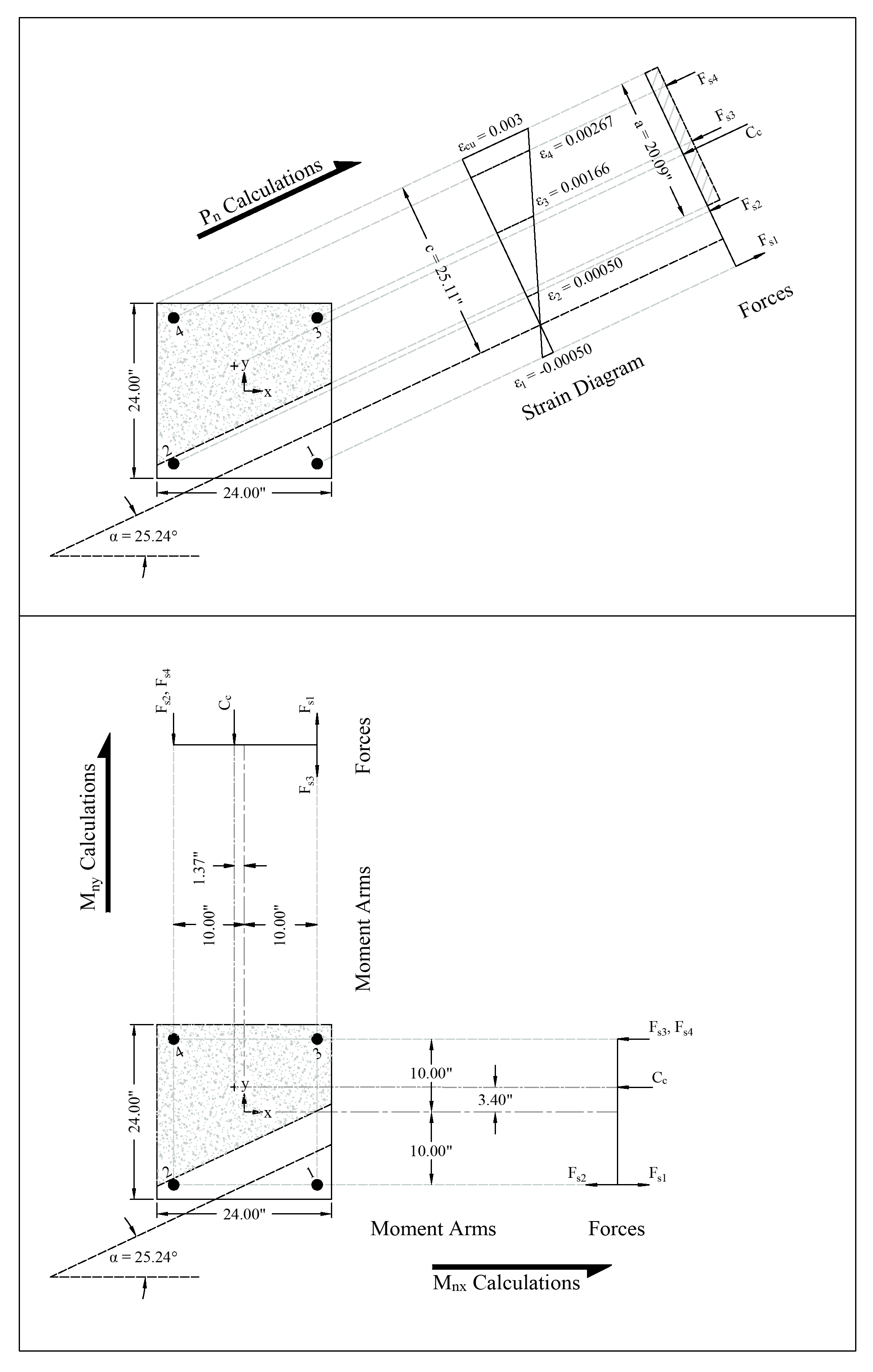
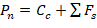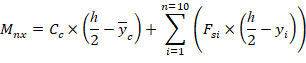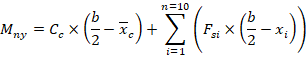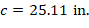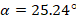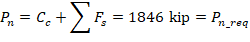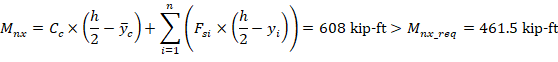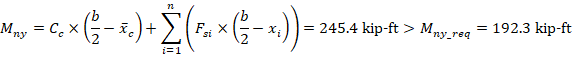4. Exact Biaxial Bending - Axial Interaction Method
In a reinforced concrete column, the exact calculations of section capacity (axial force biaxial moment strengths) involves a trial-and-error process for calculating the neutral axis depth and angle α. The steps to calculate biaxial flexural strength of a rectangular reinforced concrete column for a given nominal axial strength are as follows:
1. Assuming a value for the angle of the neutral axis (α) and the neutral axis depth (c) and calculating the strain values in each reinforcement layer
2. Calculating the forces values in the concrete (Cc) and reinforcement layers (Fsi)
3. Calculating Pn, Mnx and Mny using the following equations.
The procedure above should be repeated until the calculated Pn is equal to the given Pn.
The difficulty associated with the determination of the strength of reinforced columns subjected to combined axial load and biaxial bending is primarily an arithmetic one. The bending resistance of an axially loaded column about a particular skewed axis is determined through iterations involving simple but lengthy calculations. These extensive calculations are compounded when optimization of the reinforcement or cross-section is sought.
"Figure 19 - Column Biaxial Bending - Axial Interaction Exact Method" summarizes the exact calculations procedure to determine column section capacity subjected to axial load and biaxial bending moments. This procedure is illustrated in detail in several design examples published by StructurePoint:
“Biaxial Bending Interaction Diagrams for Square Reinforced Concrete Column Design (ACI 318-19)”
“Biaxial Bending Interaction Diagrams for Rectangular Reinforced Concrete Column Design (ACI 318-19)”
“Biaxial Bending Interaction Diagrams for C-Shaped Concrete Core Wall Design (ACI 318-19)”
“Biaxial Bending Interaction Diagrams for Spiral Reinforced Circular Concrete Column Design (ACI 318-19)”
Figure 19 - Column Biaxial Bending - Axial Interaction Exact Method